Negative Harmony sounds like a made-up term, but it’s actually a real music theory tool you can use to create interesting and unique chord progressions and melodies. However, it’s a very complex and multi-layered concept and involves a lot of calculations.
If you’ve ever heard the joke that music theory is basically just mathematics, the theory behind Negative Harmony is probably the closest you’ll get to that being true.
This post will explore in depth exactly what Negative Harmony is, how it works, and how we can use it to further our own ideas of harmony and music in general. However, let’s first take a look at what the term harmony means and how it’s created.
What is Harmony?
At its basic level, harmony in music is when there is more than one note, or pitch, sounding at the same time.
This can be two notes together – usually called an interval, or (less commonly) a dyad – or a chord, if there are three or more notes at the same time.
If melody and rhythm are related to the “horizontal” aspects of the music, then harmony deals with the “vertical” aspects.
Harmony can also refer to the entire realm of pitches and notes, and how they relate to one another in a certain piece of music.
For example, we call the system that uses twelve notes per octave “Western Harmony”, or if chords in a song are all based around one specific diatonic scale we can say that song has a “tonal harmony”.
If you want to learn more or recap about harmony, we have an article on it that goes into a lot more detail.
What is Negative Harmony?
Negative Harmony is a musical concept that is based on the work of Swiss composer and theorist Ernst Levy.
He wrote about the idea of polarity in his book A Theory of Harmony that came out in 1985, but never really gained a lot of traction in the mainstream until musician Jacob Collier repopularized it in 2017.
It is based on the inversion of chords and notes around an axis.
What Levy wrote about was the idea that in music with a tonal center (that is, any piece of music that has a key), every chord and note played has a “negative” opposite chord and note.
You can find these “negative” chords and notes by inverting (basically flipping or rotating) the regular chords of the song around an axis made by the root tonic note and the note a Perfect 5th above it.
For example, if we’re in the key of C, the axis is between the notes C — G.
The saxophonist Steve Coleman was another musician to work at developing the concept and was the first to coin the term negative harmony although composers have been toying with it going back a lot further and arguably as far back as Aristoxenus and Ptolemy!
How to Invert Notes
You might have heard of inversion with regards to intervals or chords.
With inverted intervals, the lower note of the chord or interval becomes the higher note, like a G Maj chord G-B-D would be in first inversion with the G at the top, reading B-D-G, as shown below in this chord inversion.
The G is highlighted to show how it moved from the bottom of the chord to the top.
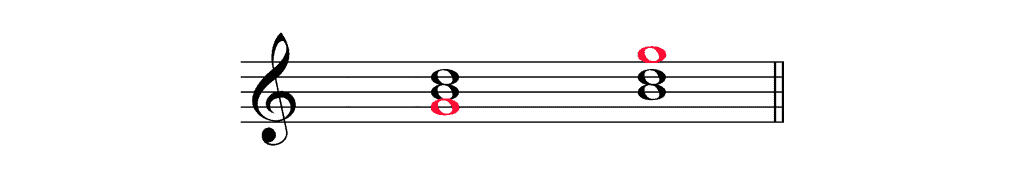
However, this is NOT what inversion means with regards to Negative Harmony.
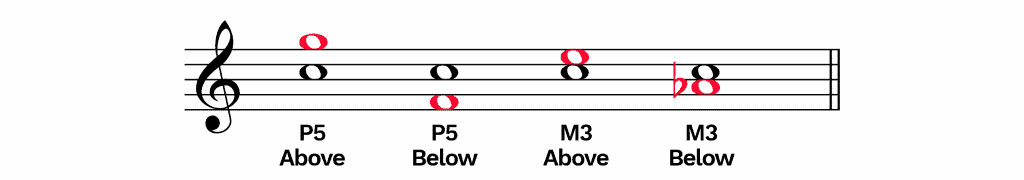
In this case, inversion means flipping or rotating around a specific axis point – taking the distance (interval) between the original note and the axis point and going that distance in the other direction.
For example, from C to G is a Perfect 5th, which is an interval of 7 semitones (half steps).
To create a Negative, or “opposite”, interval of C-G, we need to start at C and go down a Perfect 5th, or down 7 semitones.
This gets us to F, and therefore the inversion of C-G is F-C, because they have the same interval distance between them.
A Major 3rd above C would be 4 semitones up, which gets us to E.
Therefore a Maj 3rd below C would be 4 semitones below, or an Ab.
So, now that we know how to invert notes (and therefore chords), we need to determine what axis to rotate them around.
Axis of Inversion
As we mentioned above, the axis of inversion is basically a line drawn between the main tonic note of a piece of music and its Perfect 5th interval above.
In C this is C — G, in Eb it’s Eb — Bb, in F# it’s F# — C#, and so on.
But to rotate the notes, we have to do so around a specific point, not an interval, so we therefore take the exact middle point of the Perfect 5th.
Since a P5 interval is 7 semitones, the middle point would be 3.5 semitones above the low note, or below the high note.
This is always an in-between note, one that does not appear on a piano or any major instrument. In the C — G axis, this would be the point between Eb and E.
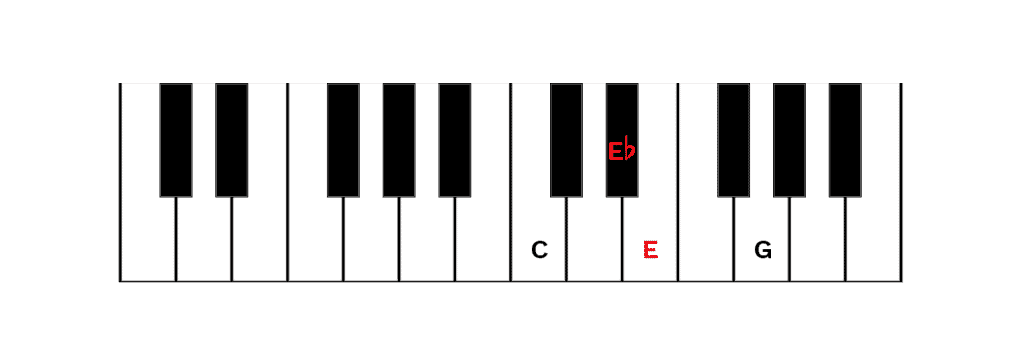
If the axis was Eb — Bb, the middle point is in between F# and G, and if the axis was F# — C#, then the middle point is between A and Bb.
To invert, you take a note below or above one of the two midpoint notes, and give it the same interval above or below the other midpoint note.
So, now we know how to rotate notes and chords via inversion, and we have a point around which to invert. But how does this actually work?
Let’s take the following melody in C Maj as an example:
This melody is C – G – F – E – A – B – C.
To create a Negative melody of these notes, each one has to be inverted around the Eb/E point – the middle of the axis created by the key center.
The first note, C, is 3 semitones lower than Eb.
Therefore to invert it, we have to go 3 semitones higher than E – to the note G.
Because C inverts to G, the second note G will then invert to C.
For the 3rd note, F, it is 1 semitone above E, and therefore will invert to 1 semitone below Eb, which is D.
The next melody note, E, is 0 semitones above E so it will invert to 0 semitones below Eb, which is still Eb.
The A and the B are 6 and 4 semitones, respectively, below the Eb, and so will invert to 6 and 4 semitones above the E – the A inverts to Bb and the B inverts to Ab.
So our new melody looks like this (G – C – D – Eb – Bb – Ab – G):
Notice how when the original melody goes up (like from C – G or A – B – C), the Negative melody goes downward, and vice versa.
Also, the intervals between each successive melody note are the same, but opposites – in the original melody, the F moves down 1 semitone to E and then jumps down a P5 to A, and the Negative melody mirrors this by moving from D up 1 semitone to Eb and then jumping up a P5 to Bb.
Here’s the list of notes and their Negative inversions around the C — G axis (E/Eb midpoint):
| A#/Bb | ⇦ Inversion (each way) ⇨ | A |
| B | ⇦ ⇨ | Ab/G# |
| C | ⇦ ⇨ | G |
| C#/Db | ⇦ ⇨ | Gb/F# |
| D | ⇦ ⇨ | F |
| D#/Eb | ⇦ ⇨ | E |
If you know the Circle of 5ths, another way to learn these inversions by mirroring the circle around a line created in between C and G.
The blue lines connect notes that are Negatives, and the red line acts as the axis of inversion.
If the song you are listening to or writing is in a key different to that of C, just rotate the Circle of 5ths diagram and draw the dotted red line between that note and its P5, and then from there connect the blue lines across it.
For example, in E, the red line would go between E — B, and F# and A would be Negative inversions, as well as Db and D, and Eb and C, etc.
Negative Chords and Chord Progressions
Hopefully you have been following along so far! This is a really tough concept to wrap your head around, and something you’ve probably never seen before.
Chords and chord progressions are even more tricky because you’re inverting multiple notes at once around the axis.
However, there are some tricks and tips you can learn to make the process easier and faster.
Just like single melody notes, chords can be inverted around an axis.
Staying in the key of C Maj, let’s say you have a chord progression that goes D min – G Maj – C Maj.
This would be ii – V – I in C, which is a very common chord progression.
So, for each chord, we have to invert every note around the E/Eb point.
The notes in D min (D – F – A) invert to become F – D – Bb, which can be written as a Bb Maj chord.
The notes in the G Maj chord (G – B – D) invert to C – Ab – F, which can be written as an F min chord.
If we keep the C chord the same (because we still want to stay in the original key of C Maj), the chord progression becomes Bb Maj – F min – C.
Just as we did with single notes, we can also use the Circle of 5ths to figure out the Negative inversions of entire chords.
Instead of drawing the axis line between C — G, we split the circle right on the C note.
The blue lines represent inverted chords, and the red dotted arrow shows a normal chord progression on the right, and the normal inverted chord progression on the left.
Also, the quality of a chord changes when it gets inverted – major chords become minor and vice versa.
So, using the diagram above, an A min chord ⇨ Eb Maj, and A Maj ⇨ Eb min.
With Seventh chords (four notes), there are also three ways they can be inverted.
Any Maj7 chord (D Maj7 = D – F# – A – C#) ⇨ Min(b6) (Bb min[b6] = Bb – Db – F – Gb)
Any min7 chord (E min7 = E – G – B – D) ⇨ Maj6 (Ab Maj6 = Ab – C – Eb – F)
Any 7 chord (G7 = G – B – D – F) ⇨ min6 (F min6 = F – Ab – C – D)
Let’s take for example this progression: iiim7 ⇨ vi7 ⇨ iim7 ⇨ V7 ⇨ I.
This is a very common chord progression, especially in Jazz and Blues music. In E Maj, this would read G#m7 ⇨ C#7 ⇨ F#m7 ⇨ B7 ⇨ E.
If we apply Negative Harmony to this progression, the new inverted progression would be:
CMaj6 ⇨ Gmin6 ⇨ DMaj6 ⇨ Amin6 ⇨ E
Summing Up Negative Harmony
The concept of Negative Harmony is a very high-level concept in music theory.
It involves a lot of transposition of notes into other notes, which we mention as inverting notes around a specific axis.
Here’s a video of Jacob Collier explaining Negative Harmony and why it creates really unique and interesting musical colors.
We hope that we were able to help you learn about Negative Harmony in this post, and that you may even be able to write out a new melody or chord progression using its techniques.

