As you hopefully know, an interval is the distance in pitch between any two musical notes.
We describe the name of the interval: 2nds, 3rds, 4ths, 5ths, etc, and the interval’s quality: major, minor, perfect, augmented, or diminished.
In this post, we’re going to look at what happens when we invert an interval and why that might be useful when working out what intervals are.
How to Invert an Interval?
To invert any interval all you need to do is take the lower note and put it above the upper note.
For example, if we had a major 3rd with C and E as shown below, we invert this interval by moving the C an octave higher so it’s above the E.
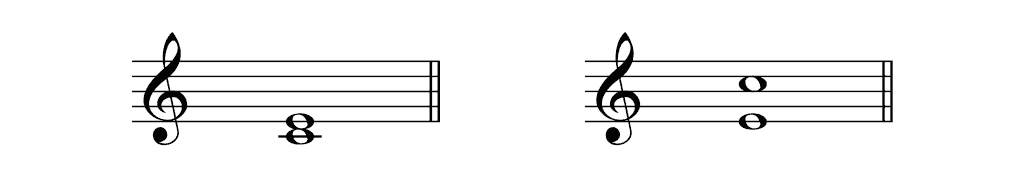
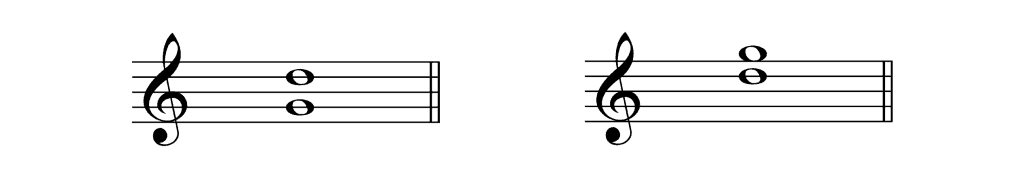
Or another example would be this perfect 5th between G and D.
If we move the G an octave higher so it’s above the D we’ve just inverted the interval.
Inverting Perfect Intervals
Perfect intervals (4ths and 5ths) have a special relationship as well.
Whenever you invert a perfect interval it becomes the opposite perfect intervals.
For example, if you were to invert a perfect 4th it would become a perfect 5th and vice versa, when you invert a perfect 5th it becomes a perfect 4th.

Perfect intervals always stay perfect unlike the next two types of intervals.
Inverting Major and Minor Intervals
Unlike perfect intervals that always stay perfect, major intervals when inverted become minor and vice versa, minor intervals when inverted become major.
For example, a major 6th when inverted becomes a minor 3rd.
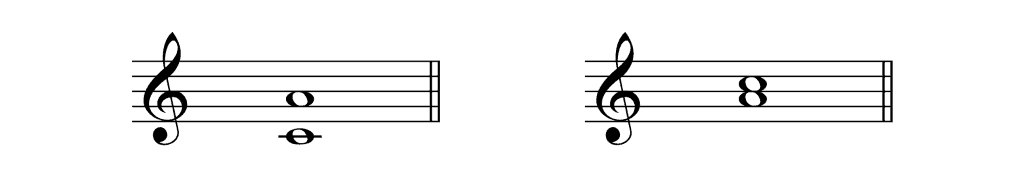
Inverting Augmented and Diminished Intervals
Augmented and diminished intervals work in a similar way to major and minor intervals.
When augmented intervals are inverted, they become diminished and vice versa, diminished intervals when inverted, become augmented.
For example, a diminished 5th when inverted becomes an augmented 4th and vice versa.
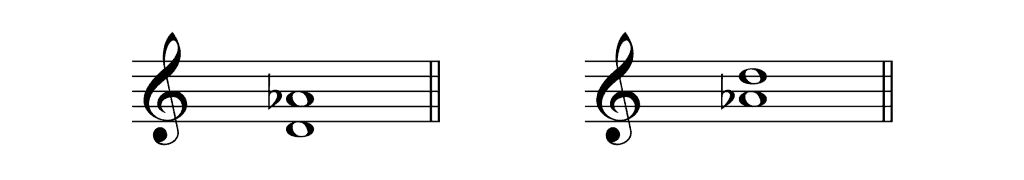
Inverted Intervals Always add up to 9
An interesting thing about inverted intervals is that when both are added together they always add up to nine.
For example, a major 3rd when inverted, becomes a minor 6th: 3 – 6 = 9.

Or a minor 2nd when inverted becomes a major 7th: 2 + 7 = 9
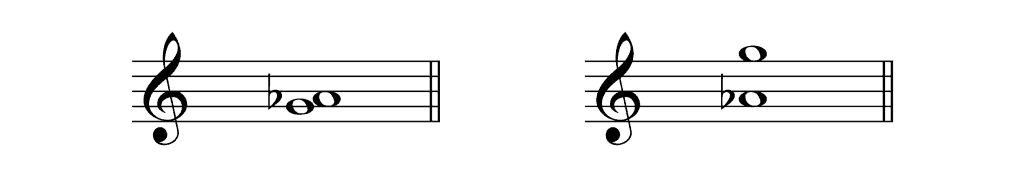
What’s the Point of Inverting Intervals?
When I first learned about inverting intervals, I was skeptical as to whether it would be useful or not, but it can come in handy when working out difficult intervals.
For example, let’s say you had to describe the interval below: E# – C.
I don’t know about you, but I’m certainly not too familiar with the E# major scale so this is will be quite a hard interval to work out.
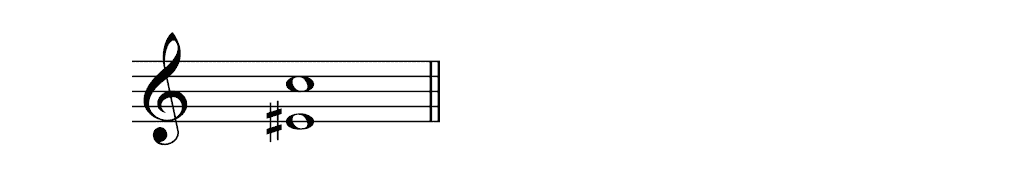
But, if we invert it, C becomes the bottom note, and I know the C major scale very well.
I can straight away see that it’s an augmented 3rd.
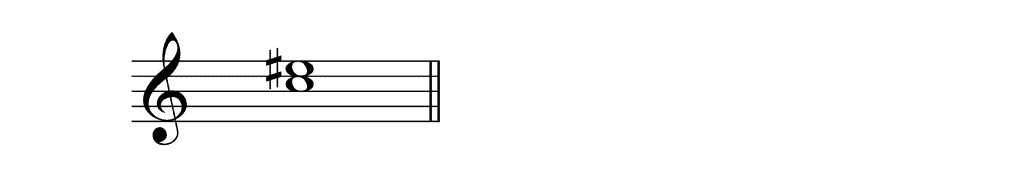
As we covered earlier when augmented intervals are inverted, they become diminished intervals.
Now I know it’s going to be some kind of diminished interval.
I also know that all inverted intervals add up to 9.
So I can ask: 3 + what = 9? It’s, of course, 6, which means that the original interval is going to be a diminished 6th.
Interval Inversion Chart
For reference, here is a chart of all the intervals when they’re inverted.
| Interval | Inverted Interval |
| Diminished 2nd | Augmented 7th |
| Minor 2nd | Major 7th |
| Major 2nd | Minor 7th |
| Augmented 2nd | Diminished 7th |
| Diminished 3rd | Augmented 6th |
| Minor 3rd | Major 6th |
| Major 3rd | Minor 6th |
| Augmented 3rd | Diminished 6th |
| Diminished 4th | Augmented 5th |
| Perfect 4th | Perfect 5th |
| Augmented 4th | Diminished 5th |
| Diminished 5th | Augmented 4th |
| Perfect 5th | Perfect 4th |
| Augmented 5th | Diminished 4th |
| Diminished 6th | Augmented 3rd |
| Minor 6th | Major 3rd |
| Major 6th | Minor 3rd |
| Augmented 6th | Diminished 3rd |
| Diminished 7th | Augmented 2nd |
| Minor 7th | Major 2nd |
| Major 7th | Minor 2nd |
| Augmented 7th | Diminished 2nd |
Conclusion
I hope that makes a bit more sense of inverted intervals and what they can be used for. Just remember that:
- Perfect intervals when inverted stay perfect
- Major intervals, when inverted become minor
- Minor intervals, when inverted become major
- Diminished intervals, when inverted become augmented
- Augmented intervals, when inverted become diminished
- All pairs of inverted intervals add up to 9



